The time value of money shows that money today is worth more than money in the future. This article shows future value and present value calculations.
This is Chapter 6 in Financial Accounting. This chapter includes:
- Time Value of Money
- Rule of 72: Formula and Calculator
- The Best Financial Calculator
- Time Value of Money YouTube playlist
For all the chapters, see The Ultimate Guide to Learn Financial Accounting
Contents
Time value of money defined
“Time is money” is a common phrase that shows the importance of the time value of money. Time value of money is a core concept in finance. Money is worth more today than it will be in the future.
Money is worth more today because of three reasons: inflation, interest, and opportunity cost.
Inflation decreases the value of money over time, so money will buy less in the future.
Money invested today can earn interest to increase its value in the future.
Opportunity cost is the cost of missing the best alternative when a choice is made with limited resources.
1. Inflation
Inflation is the decrease in the value of money. Increasing the money supply causes a reduction in the value of money. When more dollars are added to the money supply, the value of the dollar declines.
Low levels of inflation decrease money’s value slowly. Higher levels of inflation decrease the value quickly. For example, 3% inflation means that $100 at the beginning of the year would have the purchasing power of $97 at the end of the year.
At 10% inflation, $100 only buys $90 worth of goods at the end of the year.
Remember that time is money.
Benjamin Franklin
2. Interest
Interest is the charge for the use of money. Also, any return to an investor is a percentage return expressed as an interest rate. So, an investor may receive a 3% return from a bank. Another investor may earn a return on stock investments of 9.2%.
The only way for money to grow is by investing. Some returns include less risk, and some have more risk. An investment in a bank savings account is low, but there is a small risk of loss.
Stock returns can be positive or negative. So, stocks are more risky investments.
One of the basic rules in finance is that risk and return are correlated. High returns come with a high risk of loss. If the investors take small risks, the returns will also be small.
There are two ways to calculate interest:
- simple interest
- compound interest
We will discuss both simple interest and compound interest in this article.
3. Opportunity cost
Opportunity cost explains the potential benefits missed when choosing one alternative over another. In time value of money, opportunity cost is the potential return that could be earned if money was invested in an alternative over its current use.
For instance, if you have $10,000 and decide to spend it on a vacation instead of an investment. If the investment could have returned 10% annually, your opportunity cost is the forgone return or $1,000 in the first year. Additionally, the opportunity cost includes the compound interest that could have accrued over time.
Opportunity cost is the consideration of what you lose in terms of potential future value by choosing one financial decision over another.
Simple interest formula
Simple interest pays interest only on the principal, not on the accrued interest. It is the easiest interest to calculate.
The simple interest formula is Interest = Principal x Rate x Time, or I = PRT.

- P or principal, is the amount of the loan. This is listed on the loan contract.
- R, or rate, is the annual interest rate. Loans are always shown with annual percentage rates, even if the loan is for less than a year.
- T or time, is the number of days or months of the loan. This is usually a fraction of a year. So, on a 3-month note, the time is 3/12.
Simple interest is usually only calculated for one year or less. Compound interest is better for calculations over a year.
Simple interest example
Assume a $100,000 3-month note pays 12%. The interest would be I = PRT, or $100,000 x 0.12 x 3/12 = $3,000. So the borrower would borrow $100,000, and three months later he would pay $103,000.
The $103,00 equals the original $100,000 plus the accrued interest of $3,000.

Simple interest is appropriate for a single year. However, for multiple years, simple interest will earn less than compound interest.
Assume $100,000 is invested using simple interest at 10% for 5 years.
The interest would grow at $100,000 x 10% = $10,000 per year. Simple interest calculates the total interest as $50,000 for the five years. The total future value, including the principal and interest, is $150,000.
See the simple interest example below.
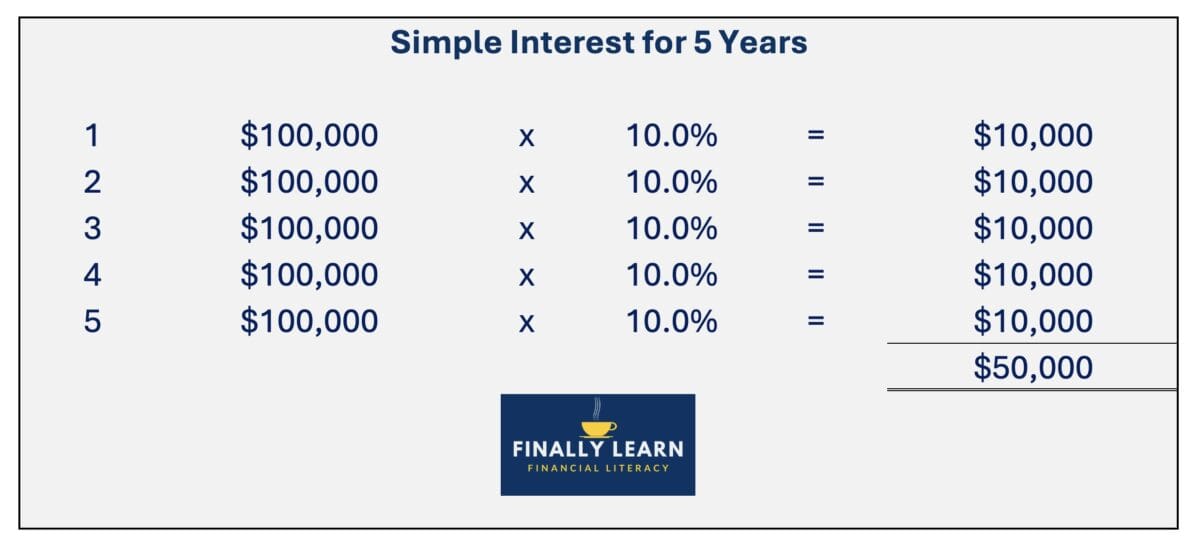
Simple interest tutorial
Simple interest is interest only on the original principal. Simple interest is useful for shorter periods.
The next section shows the advantages of compound interest.
Compound interest
Compound interest earns interest on both the principal and the accrued interest. For example, $100,000 invested using compound interest at 10% for 5 years would grow to $161,051. In the first year, the interest is $100,000 x 10% = $10,000.
Compound interest tutorial
The following video explains compound interest.
In the following years, interest is calculated on both principal and interest. See the calculations below.
So, compound interest grows faster than simple interest. Compound interest is used for interest calculations greater than a year.
Simple interest vs. compound interest tutorial
Compound interest pays interest on both principal and interest. This video shows an example of the difference between simple interest and compound interest.
Time value of money includes several formulas using compound interest:
- future value formula
- present value formula
- future value of an annuity formula
- present value of an annuity formula
- future value of an annuity due formula
- present value of an annuity due formula
These time value of money formulas use the following variables to calculate the TVM problems:
- FV: future value is a lump sum at a future date
- PV: present value is a lump sum at the current date
- r: periodic rate of return; some formulas use i for interest instead of r
- PMT: payment is an annuity or a stream of equal payments (+ or -) at equal intervals
- N: number of periods; number of years times the periods per year
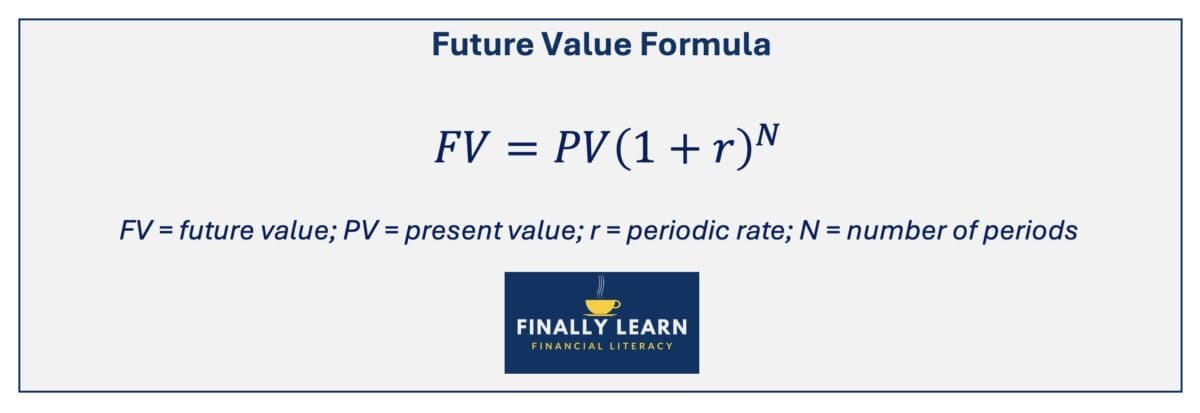
Future value formula
Future value (FV) is the value of an investment at a future date. The FV formula calculates the growth of a lump sum over time using a constant rate of return. Growing an investment into the future is called compounding.
The future value of a lump sum formula is:
Future value example
Let’s use the same problem as the simple interest above. Assume $100,000 is invested using compound interest at 10% for 5 years.

The $100,000 investment today grows to $161,051 using compound interest. Remember, the simple interest future value was only $150,000.
| Interest | Future Value |
|---|---|
| Simple Interest | $150,000 |
| Compound Interest | $161,051 |
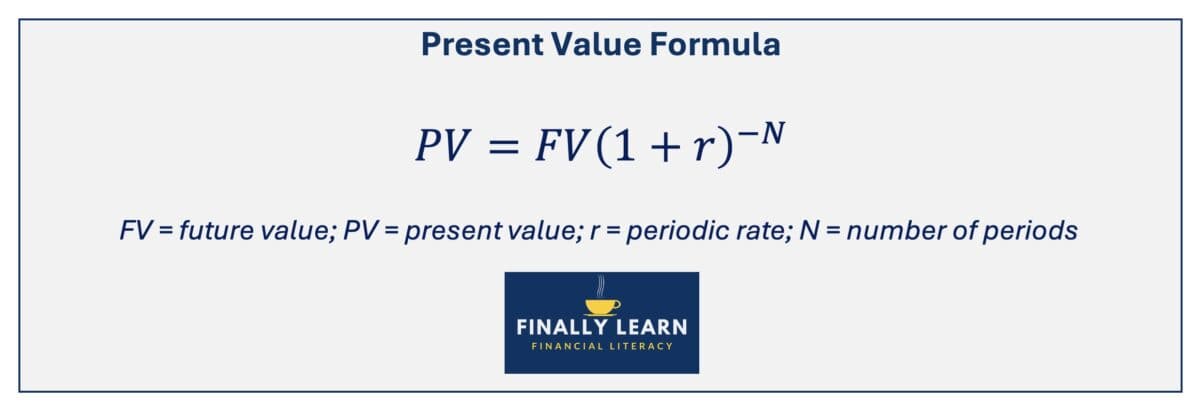
Present value formula
Present value (PV) is the value of an investment at present. The PV formula calculates the current value of a future lump sum over time using a constant discount rate. Calculating a future value in current dollars is called discounting.
The present value formula is:
Present value example
Assume $100,000 will be received at the end of 5 years. What is the present value if the discount rate is 10%?

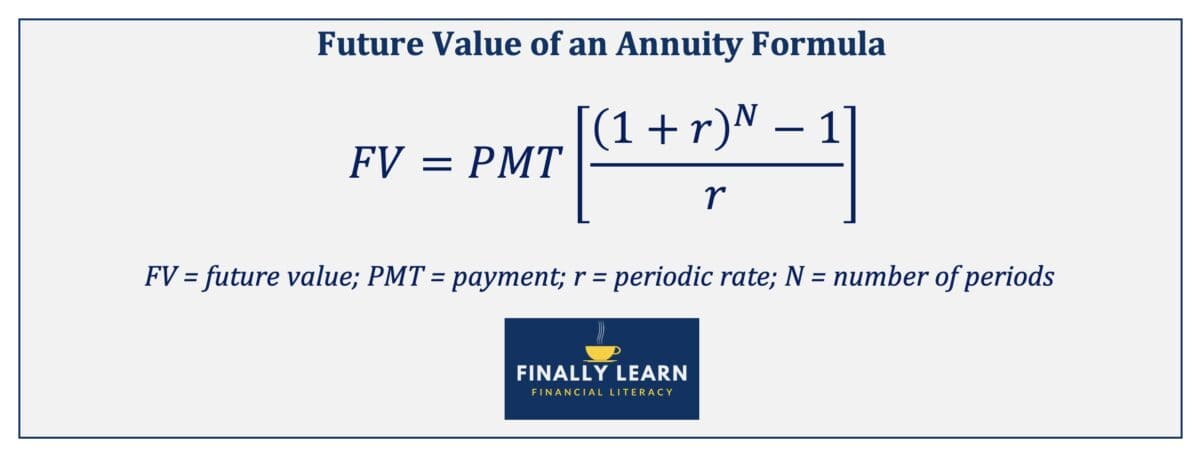
Future value of an annuity
An annuity is a stream of equal payments or receipts at equal intervals. If the payments occur at the end of the period, it is an ordinary annuity. If the payments occur at the beginning of the period, it is an annuity due.
Here is the future value of an ordinary annuity formula:
Future value of an annuity example
Assume $10,000 is invested annually for 5 years. The interest rate is 10%. What is the future value of the ordinary annuity?
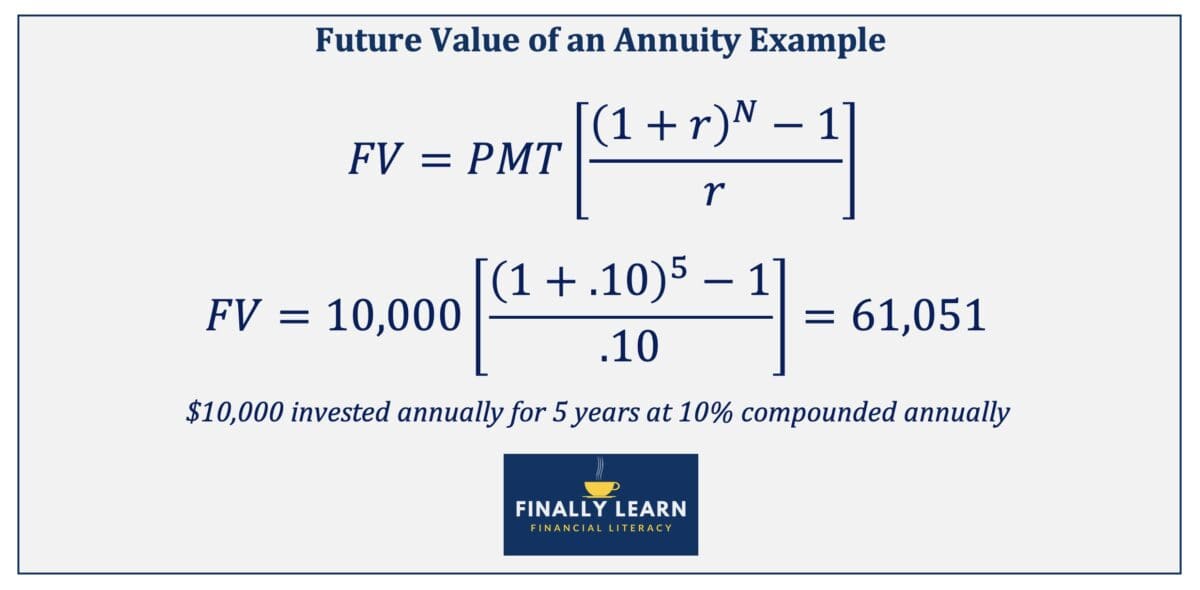
The future value of the annuity is $61,051.
Present value of an annuity
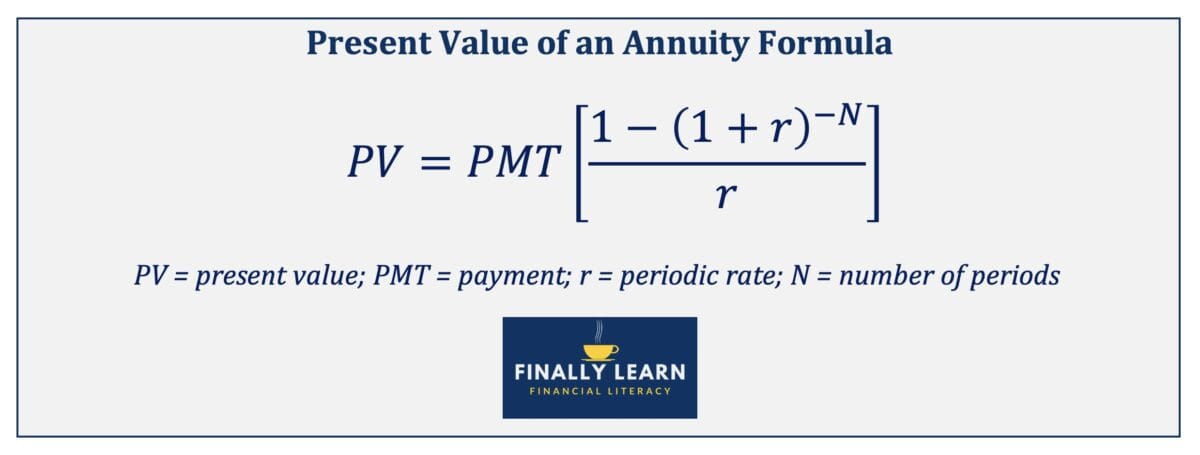
Present value of an annuity example
Assume $10,000 payments occur annually for 5 years. If the discount rate is 10%, what is the present value of the annuity?
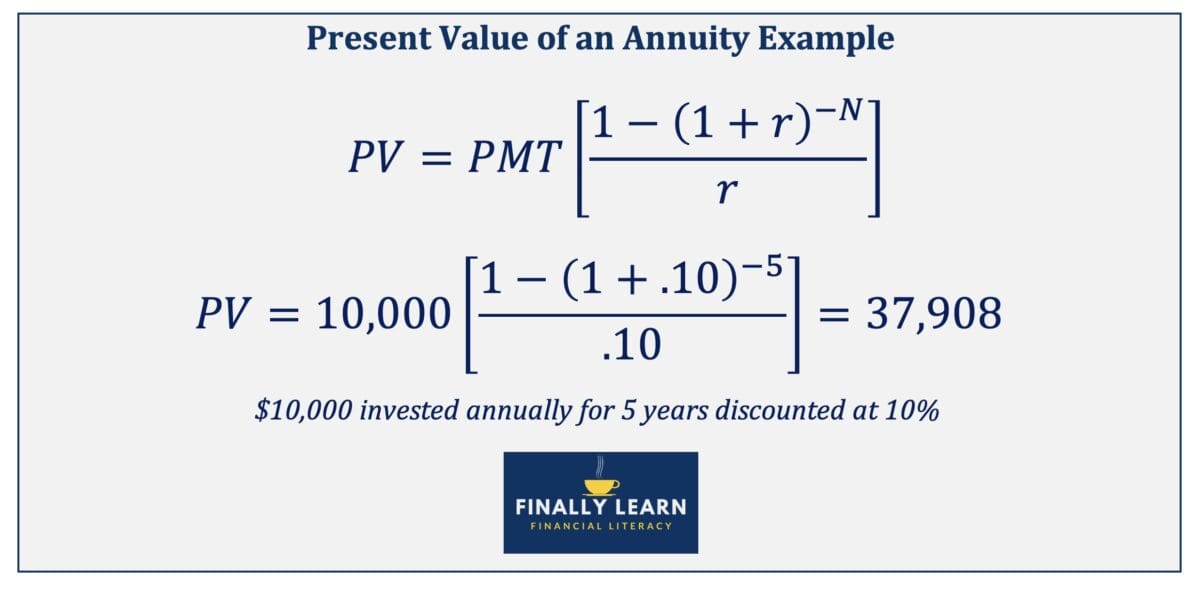
The present value of the annuity is $37,908.
Time value of money using Excel
The time value of money can be calculated using several different methods:
- formulas
- Excel spreadsheets
- financial calculators
- interest tables
Time value of money using Excel tutorial
Time value of money resources
- Harvard Business School: Time Value of Money (TVM): A Primer
- Sullivan University: Time Value of Money
- FN calculator: Financial calculators
- Finally Learn: Financial Functions in Excel
Accounting Chapters
Here are the accounting chapters in The Ultimate Guide to Learn Accounting:
- Introduction to Accounting
- Recording Business Transactions
- Adjusting Entries and the Accounting Cycle
- Accounting for Merchandising Activities
- Inventory and Cost of Goods Sold
- Cash and Internal Control
- Accounting for Receivables
- Accounting for Long-Term Assets
- Accounting for Current Liabilities
- Accounting for Long-Term Liabilities
- Corporations
- Statement of Cash Flows
- Financial Statement Analysis
- Managerial Accounting
- Job Order Costing
- Process Costing
- Activity Based Costing
- Cost Volume Profit Analysis
- Variable Costing
- Master Budgets
- Standard Costing
- Performance Measurement
- Relevant Costing
- Capital Budgeting
- Time Value of Money
See also Accounting Sample Exams.




